Level 1 CFA® Exam:
Probability – Practical Problems
In this lesson, we're going to distinguish between conditional and unconditional probability and define the basic rules of probability. We're also going to talk about dependent and independent events and solve a few probability problems.
Let's start with conditional and unconditional probability. When we want to find the probability of an event, we're simply trying to determine its unconditional probability. The unconditional probability of event A is denoted P(A) and is sometimes referred to as marginal probability.
Usually, the more information we have or the more restrictions we set, the more accurately can determine the probability of an event. Imagine that there are two events A and B, and we know the relationship between the probabilities of these events, for example, we know the probability that both events occur. If it is the case, we can more accurately estimate the probability of these events. This is when we're dealing with conditional probability, expressed in the following way:
The probability of event A, given that event B has occurred, equals the ratio of the joint probability of events A and B and the probability of event B.
Just to remind you, by "joint probability of events A and B" we understand the occurrence of both events A and B.
Of course, the probability of event B has to be greater than 0.
The probability that real GDP increases in the third quarter is 0.8. The probability that real GDP increases in the third quarter and Loop Fund achieves a higher return than the S&P 500 in the third quarter is 28%.
What is the probability that Loop Fund outperforms S&P 500, given that the increase in real GDP in the third quarter occurs?
(...)
Now we're going to discuss three rules that you need to know when solving probability problems. The rules apply to different kinds of probability, both objective and subjective ones.
We're talking about:
- the multiplication rule for probabilities,
- the addition rule for probabilities, and
- the multiplication rule for independent events.
To calculate a conditional probability we need the joint probability of two events. The multiplication rule for probabilities states that:
The joint probability of events A and B equals the probability of event A given event B occurs multiplied by the probability of event B.
The multiplication rule for probabilities is useful when we know the conditional probability and want to find the joint probability.
What is the probability that events A and B occur if the probability of B given A equals 0.4 and the probability of A is 0.7?
We need to use the multiplication rule for probabilities:
\(P(AB)=P(B|A)\times P(A)=0.4\times0.7=0.28\)
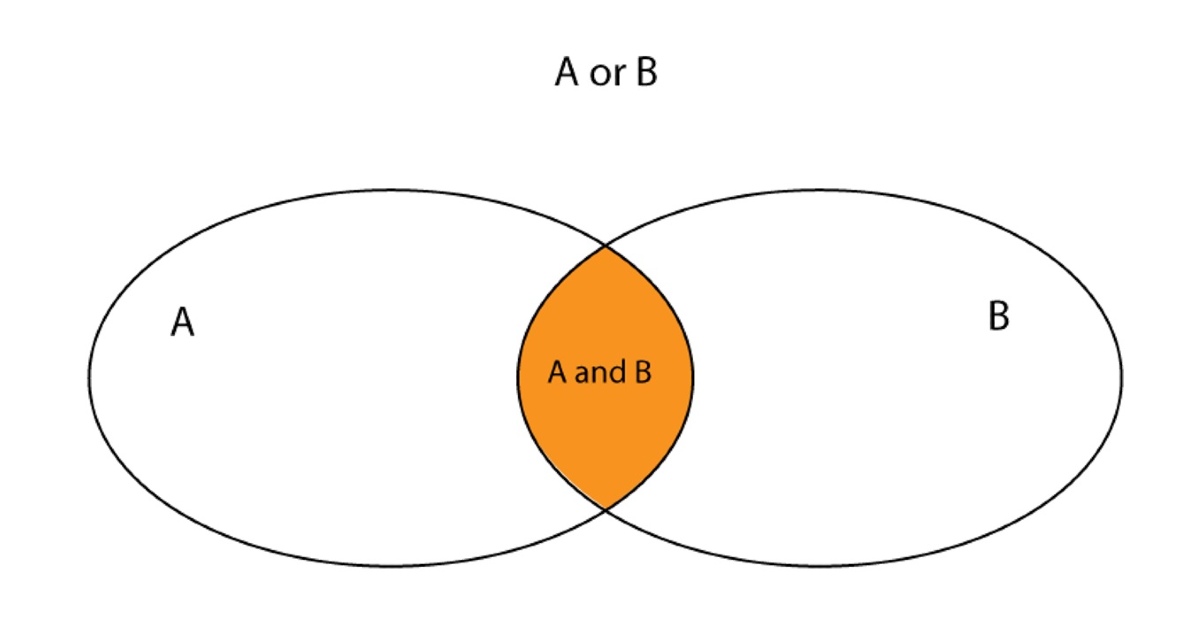
The addition rule for probabilities allows us to determine the probability that event A or B occurs, or that both A and B occur. It can be represented with this expression:
The probability that at least one of the two events A and B occurs equals the probability of event A plus the probability of event B minus the joint probability of events A and B.
Why do we subtract the joint probability?
We subtract the joint probability because if we don’t do this, we will use it in our calculation twice. Look at the following example.
We roll a dice once:
- Event A occurs when we roll 1 or 2 or 3.
- Event B occurs when we roll 3 or 4 or 5.
What is the probability that event A or B occurs?
\(P(A)=\frac{3}{6}=0.5\)
\(P(B)=\frac{3}{6}=0.5\)
If we calculated the probability that at least one of the two events A and B occurs as the sum of event A and event B, that is 0.5 plus 0.5, we would get a probability equal to 1. We know well that it can’t be correct because neither of the events includes rolling a 6.
So, to calculate the probability that at least one of the two events A and B occurs, we first have to calculate the joint probability of events A and B. Because the only outcome that occurs in both events is 3, this probability is equal to:
\(P(AB)=\frac{1}{6}\)
So, the probability that event A or B occurs equals:
\(P(A\cup{B})=P(A)+P(B)-P(AB)=\frac{3}{6}+\frac{3}{6}-\frac{1}{6}=\frac{5}{6}\)
Note that when the joint probability of A and B equals 0, that is the events don’t occur at the same time, the following holds:
The probability that at least one of the two events A and B occurs equals the sum of the probability of event A and the probability of event B.
Also, if one of the events – for example event A – contains the other – that is event B, the joint probability of events A and B equals the probability of event B. Moreover, the probability that event A or B occurs equals the probability that event A occurs.
An investment company has a team of eight analysts specializing in equity instruments and six specializing in debt instruments. A total of 20 analysts work for the company, two of whom have a reputation as experts in both equity and debt instruments. What's the probability that a randomly selected analyst will be an expert in equity instruments or debt instruments or both types of instruments at the same time?
(...)
A dependent event is an event whose probability changes depending on the occurrence of other events. An independent event is an event whose probability is not affected by whether or not another event occurs. Events A and B can be said to be independent only if the following equivalent equations hold:
The joint probability of events A and B equals the probability of event A times the probability of event B. Also, these relationships hold for independent events A and B:
- the probability of event A, given event B has occurred, equals the probability of event A, and
- the probability of event B, given event A has occurred, equals the probability of event B.
(...)
Remember that two or more events can be either dependent or independent. If events are not INDEPENDENT, they MUST be DEPENDENT.
The probability of event A equals 0.8, the probability of event B equals 0.6 and the probability of event C equals 0.3. The events are independent. What's the joint probability of these three independent events?
Based on the multiplication rule for independent events, we can tell that the joint probability equals:
\(P(ABC)=P(A)\times{P(B)}\times{P(C)}=0.8\times0.6\times0.3=0.144\)
So, the probability that all three events occur is equal to 14.4%.
- The unconditional probability of event A is denoted P(A) and is sometimes referred to as marginal probability.
- To calculate a conditional probability we need the joint probability of two events.
- The multiplication rule for probabilities is useful when we know the conditional probability and want to find the joint probability.
- The addition rule for probabilities allows us to determine the probability that event A or B occurs, or that both A and B occur.
- A dependent event is an event whose probability changes depending on the occurrence of other events. An independent event is an event whose probability is not affected by whether or not another event occurs.
- The joint probability of N independent events is the product of the individual probabilities of the events.
- Two or more events can be either dependent or independent. If events are not INDEPENDENT, they MUST be DEPENDENT.