How to Use Matrix Pricing in Your Level 1 CFA® Exam?

This blog post was created as a part of the CFA exam review series to help you in your level 1 exam revision, whether done regularly or shortly before your CFA exam.
Matrix Pricing – Definition
If we have the data for bonds with different maturities and similar credit risks that are actively traded on the market and want to compute the price of a bond that is not actively traded, we can use matrix pricing.
Generally, we use matrix pricing in two cases:
- either when we want to value a bond that is illiquid, or
- when we want to find the required yield spread for a bond that is to be issued in the near future.
Matrix Pricing Algorithm for Illiquid Bonds
If we have the data for bonds with different maturities and similar credit risks that are actively traded on the market and want to compute the price of a bond that is not actively traded, we can use matrix pricing.
The algorithm is as follows:
- Compute the average YTM for each maturity for actively traded bonds.
- Estimate the market discount rate for a bond that is not actively traded using linear interpolation and the YTMs from Step 1.
- Compute the price of the illiquid bond using the yield-to-maturity (YTM) computed in Step 2.
In Question 1 below, we show how to use this algorithm in your level 1 CFA exam.
The algorithm is very similar when we apply matrix pricing to calculate the required yield spread (it also uses linear interpolation). The application of the algorithm is presented in Question 2 below.
The table gives the information about six bonds:
Bond | Maturity | YTM |
A | 4 | 5% |
B | 4 | 5.5% |
C | 4 | 6% |
D | 7 | 7.6% |
E | 7 | 7.9% |
F | 7 | 8.2% |
An investor wants to value a six-year Bond X with a par value equal to USD 100 that pays coupons annually. The coupon rate equals 5%. Assuming that the bonds given in the table and Bond X are characterized by a similar credit risk, the YTM for Bond X is closest to:
- 5.5%.
- 7.1%.
- 7.9%.
Answer: B
We will use matrix pricing to estimate the market discount rate.
The algorithm is as follows:
- Compute the average yield for four-year bonds and seven-year bonds.
- Estimate the market discount rate for the six-year bond using linear interpolation.
The average yield for four-year bonds:
YTM_4=\frac{5\%+5.5\%+6\%}{3}=5.5\%
The average yield for seven-year bonds:
YTM_7=\frac{7.6\%+7.9\%+8.2\%}{3}=7.9\%
Now we estimate the six-year market discount rate using linear interpolation:
YTM_6=5.5\%+\frac{6-4}{7-4}\times{(7.9\%-5.5\%)}=7.1\%
As you can see the YTM for the six-year bond is between 5.5% (the average YTM for a four-year bond) and 7.9% (the average YTM for a seven-year bond).
As soon as we have the YTM, we can compute the price of Bond X. We won’t do that, however, because that’s not what the question asks us about. Also note that in this particular example, we could have just as well chosen the right answer, i.e. 7.1%, without calculating the exact YTM for Bond X (the average yield for four-year bonds amounted to 5.5%, i.e. answer A, whereas for seven-year bonds to 7.9%, i.e. answer C).
In the table, you can find information about two Treasury bonds:
Bond | Maturity | YTM |
S | 3 | 1.5% |
T | 6 | 4.5% |
Active Saturday, Inc. wants to issue a five-year bond. The rate of return required by investors on the four-year bond issued by the company is 3.9%. What is the required yield spread over the benchmark rate on the five-year bond that the company is going to issue?
Assume that the yield spread is the same for all maturities.
- ...
- ...
- ...
Question 2 is available for CFA candidates using our study planner to control their prep:
Already using Soleadea? 1. Sign into your account 2. Refresh this page to see Question 2.
Level 1 CFA Exam Takeaways for Matrix Pricing
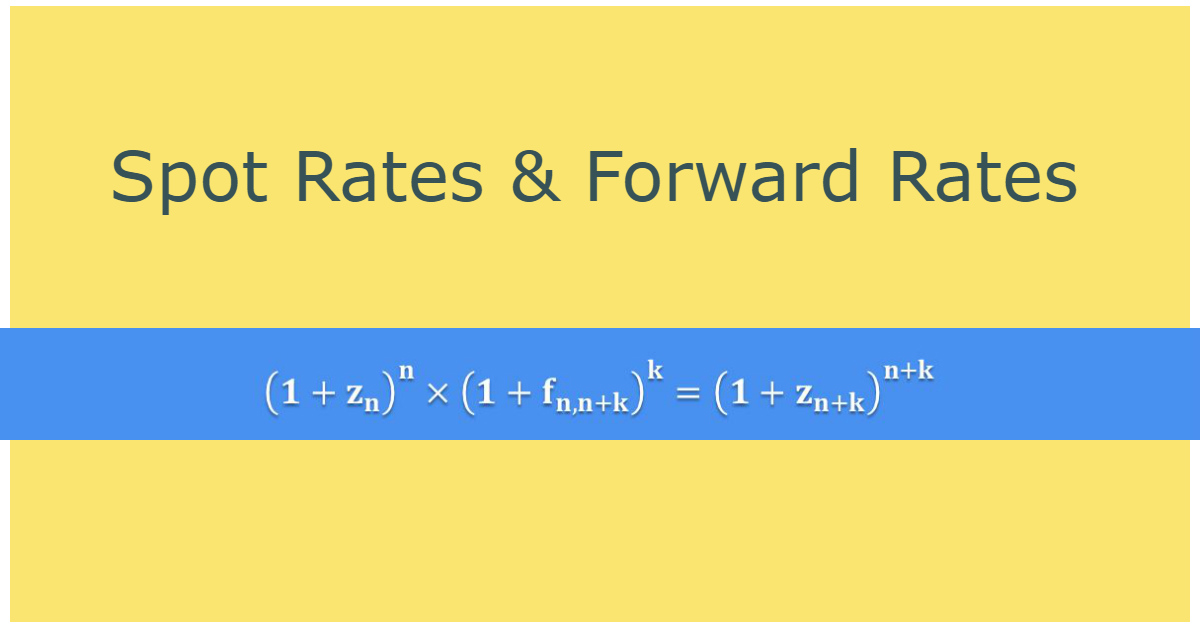
- There are different methods and techniques used in the bond valuation process. We can value a bond using: a market discount rate, spot rates and forward rates, binomial interest rate trees, or matrix pricing.
- Matrix pricing is an estimation process we use when we don’t know the market discount rate for a particular bond.
- Generally, we use matrix pricing in two cases: either when we want to value a bond that is illiquid (not actively traded on the market), or when we want to find the required yield spread for a bond that is to be issued in the near future.
- In both cases, we use linear interpolation and the yields to maturity of bonds with similar credit ratings (risks) to compute the market discount rate.
LAST UPDATE: 2 Nov 2023
Read Also: